At What Nominal Rate Compounded Continuously Must Money Be Invested to Triple in 6 ‹years
Nominal Interest Rate
The rate of interest before adjusting for inflation
What is the Nominal Interest Rate?
The nominal interest rate refers to the rate of interest before adjusting for inflation. It also refers to the rate specified in the loan contract without adjusting for compounding. The nominal interest rate is in contrast to the real interest rate regarding the inflation adjustment and effective interest rate regarding the compounding adjustment.

Nominal interest rates can be impacted by different factors, including the demand and supply of money, the action of the federal government, the monetary policy of the central bank, and many others.
Central banks implement the short-term nominal interest rate as a tool of monetary policy. During an economic recession, the nominal rate is lowered to stimulate economic activities. During inflationary periods, the nominal rate is raised.
Summary
- In contrast to the real interest rate, the nominal interest rate refers to the rate of interest before adjusting for inflation.
- According to the Fisher Effect, nominal interest rate equals real interest rate plus the expected inflation rate.
- In contrast to effective interest rate, the nominal interest rate refers to the rate specified in the loan contract without adjusting for compounding.
Nominal Interest Rate vs. Real Interest Rate
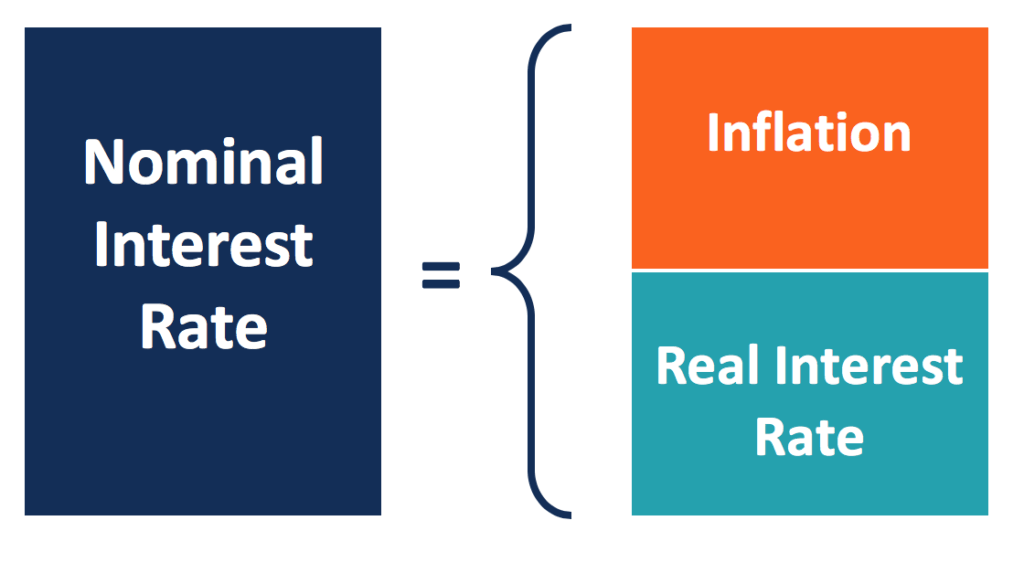
Interest rate is the cost of borrowing or return of lending due to the time value of money. The rate is known as the nominal rate, which is stated in the loan contract. A nominal interest rate contains two parts: a real interest rate and an inflation premium.
As an economy grows with inflation, the purchasing power of each dollar declines over time. Thus, the return that a lender earns for each dollar he lent before is actually lower than the rate stated in the contract. The rate of return after adjusting the nominal interest rate for inflation is known as the real interest rate. It is important for a lender to understand the real interest rate of a bond.
Inflation Adjustment of Nominal Interest Rate
The Fisher Effect describes the relationship between inflation and nominal or real interest rate through the equation below:
(1 + i) = (1 + R) (1 + h)
Where:
- i – Nominal interest rate
- R – Real interest rate
- h – Expected inflation rate
In a stable economy that is growing at a moderate pace, the inflation rate is usually low. With a low inflation rate, a simplified version of the Fisher equation can be implemented. It states that the nominal interest rate is approximately equal to the real interest rate plus the inflation rate (i = R + h).
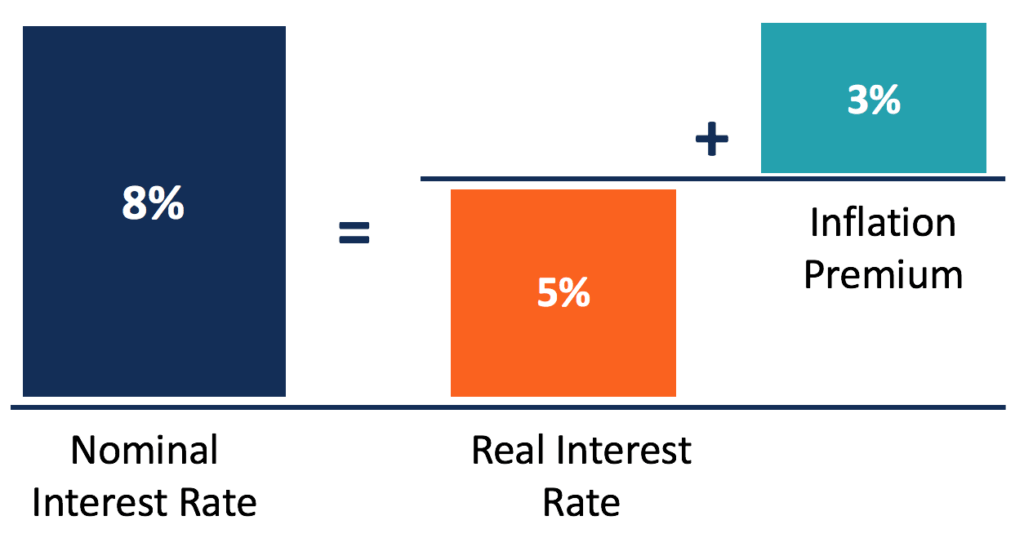
For example, a bond investor is expecting a real interest rate of 5%, when the market shows an expected inflation rate of 3%. Therefore, the investor should look for a bond with a stated (nominal) interest rate of 8% (5% + 3%).
The nominal interest rate can also be calculated through the formula below. The two methods of calculation give a similar result.
i = (1 + R) (1 + h) – 1 = (1 + 5%) (1 + 3%) – 1 = 8.15%
According to the Fisher Effect, if the inflation rate increases and the nominal interest rate remains constant, the real interest rate will fall. The lender's real return drops as a result of a faster decline in the purchasing power. If the nominal interest rate and expected inflation rate both increase at the same rate, which means the inflation premium is compensated, the real interest rate will remain unchanged.
Nominal Interest Rate vs. Effective Interest Rate
The nominal interest rate refers to the periodic interest rate multiplied by the number of periods in a year. It does not take compounding into account. Therefore, nominal interest rates are not always comparable, unless they include the same compounding periods.
Effective interest rate is considered a more accurate measure of interest. It is calculated based on the nominal interest rate and its compounding periods. Different effective interest rates can be compared directly since they've been adjusted to reflect the effect of compounding.
Compounding Adjustment of the Nominal Interest Rate
The relationship between a nominal rate and an effective rate with discrete compounding period adjustment is shown below:
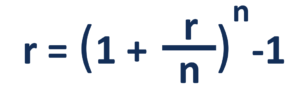
If the interest is compounded continuously,
![]()
Where:
- i – Nominal interest rate
- r – Effective interest rate
- n – Number of compounding periods per year
- e – Natural log base (2.71828…)
The nominal and effective interest rates are not always different. If the compounding period is exactly one year, the nominal rate and effective rate will be the same. Consider a bond with an annual interest rate of 5%, compounded annually. The nominal interest rate of the bond is 5% (with a periodic rate of 5% and one compounding period per year). The effective interest rate is also 5%.
As long as the compounding period is less than one year, the effective interest rate is greater than the nominal interest rate. At the same nominal rate, the shorter the compounding period (more compounding periods per year), the greater the effective interest rate is, and the continuously compounded bond offers the highest effective rate.
In the example above, the effective rate will be 5.095% if the 5% interest is compounded quarterly, 5.116% for monthly compounding, and 5.127% for continuous compounding. The calculation is shown below:
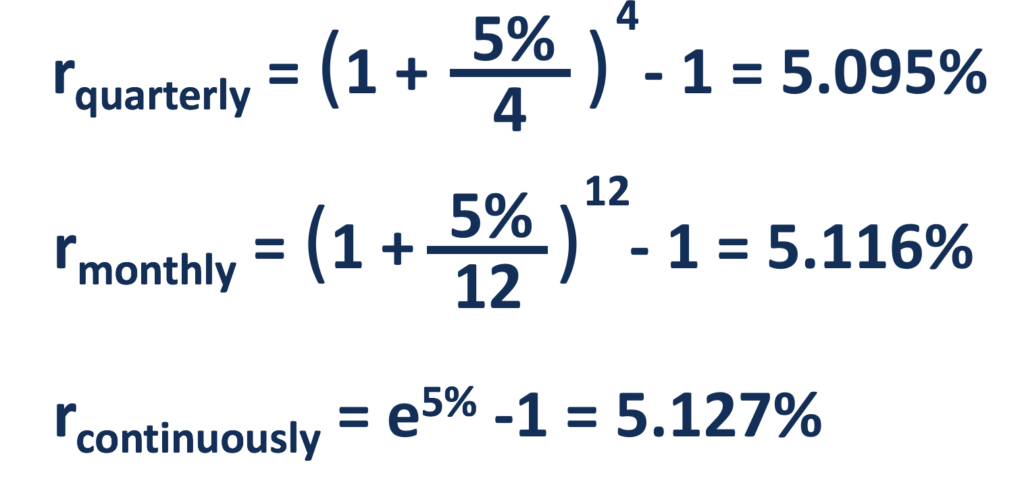
Additional Resources
Thank you for reading CFI's guide on Nominal Interest Rate. To keep advancing your career, the additional resources below will be useful:
- Applicable Federal Rate
- Continuously Compounded Interest
- Effective Annual Interest Rate
- Recession
Source: https://corporatefinanceinstitute.com/resources/knowledge/finance/nominal-interest-rate/